eo_logo
 
Product added to cart

 
Gaussian Beams Calculator

View All Technical Tools

Axial Distance, $ z  \left[ \text{mm} \right] $:
Beam Waist, $ \omega_0  \left[ \text{mm} \right] $:
Wavelength, $ \lambda [ \large{\unicode[computer modern]{x03BC}} \text{m} ] $:

Half Beam Diameter, $ \omega \! \left( z \right) \left[ \text{mm} \right] $: --        

Radius of Curvature, $ R \! \left( z \right) \left[ \text{mm} \right] $: --        

Rayleigh Range, $ Z_R  \left[ \text{mm} \right] $: --        

Rayleigh Half Diameter, $ \omega_R \left[ \text{mm} \right] $: --        

Half Angle Divergence, $ \theta \left[ \text{mrad} \right] $: --        

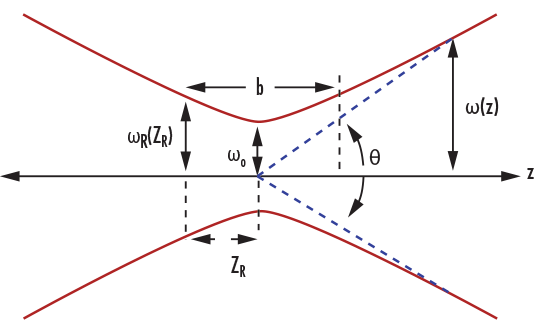
Gaussian Beam Calculator

$$ z_R = \frac{\pi \omega_0 ^2}{\lambda} $$

$$ \omega \! \left( z \right) = \omega_0 \sqrt{1 + \left( \frac{z}{z_R} \right) ^2} $$

$$ Z_R = \frac{b}{2} $$

$$ \omega_R = \omega \! \left( Z_R \right) = \sqrt{2} \cdot \omega_0 $$

$$ R \! \left( z \right) = z \left[ 1 + \left( \frac{z_R}{z} \right)^2 \right] $$

$$ \theta = \frac{\lambda}{\pi \, \omega_0} $$
$$ \lambda $$ Wavelength
$$ Z_R $$ Rayleigh Range
$$ z $$ Axial Distance
$$ \omega \! \left( z \right) $$ Half Beam Diameter
$$ \omega_0 $$ Beam Waist
$$ b $$ Confocal Parameter
$$ \omega_R $$ Rayleigh Half Diameter
$$ R \! \left( z \right) $$ Radius of Curvature
$$ \theta $$ Half Angle Divergence
Notes:
  • Please use the American standard for number formatting rather than the European standard (i.e. for "two and a half," enter "2.5" rather than "2,5").
  • Results greater than 1,000,000 are rounded to infinity.

Description

Mathematically model beam propagation of Gaussian beam using simple geometric parameters. Calculator uses first-order approximations and assumes TEM00 mode to determine beam spot size in free space applications. Please note that results will vary based on beam quality and application conditions.

Related Resources and Products

 Application Note
Gaussian Beam Propagation
Gaussian Beam Propagation


 Application Note
Fundamental of Lasers
Fundamental of Lasers


 Application Note
Beam Expanders
Beam Expanders


 FAQ
I thought beam expanders increased a laser beam's size. How can that help me to get a smaller spot?
I thought beam expanders increased a laser beam's size. How can that help me to get a smaller spot?

Related Products

Was this content useful to you?
 
Sales & Expert Advice
 
or view regional numbers
 LIVE CHAT
Easy-to-Use
QUOTE TOOL
enter stock numbers to begin
Sign-up Today // industry trends, new products, discounts, and technical content Sign-up Now
About Us Company Executive Team Tradeshows Regional Offices Careers Press Releases Case Studies Compliance TECHSPEC® Brand Partnerships Contact Us
Customer Care Shipping Returns FAQs Give Feedback Manufacturing Services Precision Optics Optical Filters Laser Optics Imaging Optics Defense Optics
Discounts & Programs All Discount Programs Vision Integration Partners Volume Discount Services Educational Discount Program Educational Award Discounted Products Edmund Scientific Outreach E-procurement Platforms
Catalogs All Catalogs Digital Catalogs Catalog Unsubscribe We are Hiring! Apply Now

Copyright 2023, Edmund Optics India Private Limited, #267, Greystone Building, Second Floor, 6th Cross Rd, Binnamangala, Stage 1, Indiranagar, Bengaluru, Karnataka, India 560038

Privacy Policy | Cookie Policy | Terms & Conditions | Accessibility
California Consumer Privacy Acts (CCPA): Do Not Sell or Share My Personal Information
California Transparency in Supply Chains Act

ISO Logo PJR Logo itar Logo Secure Trust Logo Women Owned Business